Now I will demonstrate the calculation of the value necessary for the formula above.

The determination of the concentration of the particles on the surface of the sun is possible by means of temperature of the surface. This determination will be effected in the following auxiliary calculation:

The concentration of the sun-particles can be derived from photons entering per unit area and their duration of stay in the volume of 1m³. It is assumed that the photons are independent of each other and that three sun-light particles belong to every photon according to the definition of l. The number of entering photons is calculated from the following equation according to the number /3/ of the references.

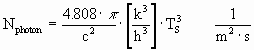
The number of sunlight-particles within a volume of 1 m³ can be derived from the number of entering sunlight-particles and their duration of stay.

When photons enter a plane of 1m² the duration of stay t can be determined for a volume of 1m³ by using the distance of 1m.

It follows:

The temperature T_S on the surface of the sun can be calculated applying the solar-constant (1395 W/m²) and the energy E of electrmagnetically radiation as a function of the temperature by using the law of radiation by STEFAN-BOLTZMANN

The term E is calculable using the distance between the earth and the sun with the term d_E = diameter of the earth:

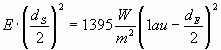au=astronomic unit

hence it follows: T_S = 5806 K and

(end of auxiliary calculation)

With the following equation all values are available for the calculation of a:

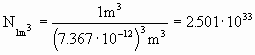
a=1.49*10^-4 grd

Now one obtains the value of deflection of the star-light:

a = 0.535"      instead of  1.75"       deviation: -70%

The deviation is high and I have not found a cause for that fact. The consideration of the neutrinos coming from the sun as equivalent to the sun-light-particles is not enough to prevent the deviation. This would be only possible with a substantial higher number of neutrinos.

next page

previous page

list of contents

back to the start